Vrijeme: 22:17
Perspektivni lanac | A chain with perspective #2
Slikar i dalje proučava perspektivu. Zna da se pravci koji su paralelni u prostoru, kad se crtaju u perspektivi, na slici svi sijeku u jednoj točki, točki nestajanja. Odredite gdje je na staklu točka nestanja skupa svih pravaca paralelnih s pravcem koji prolazi točkama 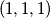 i
i  . Staklena ravnina se nalazi u
. Staklena ravnina se nalazi u 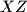 ravnini, a slikarovo oko u točki
ravnini, a slikarovo oko u točki 
Odgovor napišite kao uređenu trojku koordinata, npr. "(1,2,3)", bez navodnika.
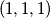 i
i  . Staklena ravnina se nalazi u
. Staklena ravnina se nalazi u 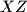 ravnini, a slikarovo oko u točki
ravnini, a slikarovo oko u točki 
Odgovor napišite kao uređenu trojku koordinata, npr. "(1,2,3)", bez navodnika.
The artist is continuing to further his understanding of perspective. Knowing that, when drawn in perspective, any set of lines parallel in space intersect at one point on the painting, the vanishing point. Determine the vanishing point on the pane of glass of the set of all lines parallel to the line witch passes through the points 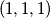 and
and  . The glass pane is positioned to match the
. The glass pane is positioned to match the 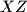 plane and the artist's eye is at
plane and the artist's eye is at 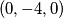 .
.
Write the answer as an ordered triple of coordinates, e.g. '(1,2,3)' without quotes.
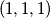 and
and  . The glass pane is positioned to match the
. The glass pane is positioned to match the 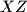 plane and the artist's eye is at
plane and the artist's eye is at 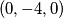 .
. Write the answer as an ordered triple of coordinates, e.g. '(1,2,3)' without quotes.
