Vrijeme: 05:41
Njemački lanac | German chain #1
Allice (A) i Bob (B) svađali su se oko pravaca jednakog smjera. Mogu li ikada sječi? Prvo su odlučili pokušati dilemu riješiti na listu papira i saznali su da se dva pravca ili nikada ne sijeku ili su identični. 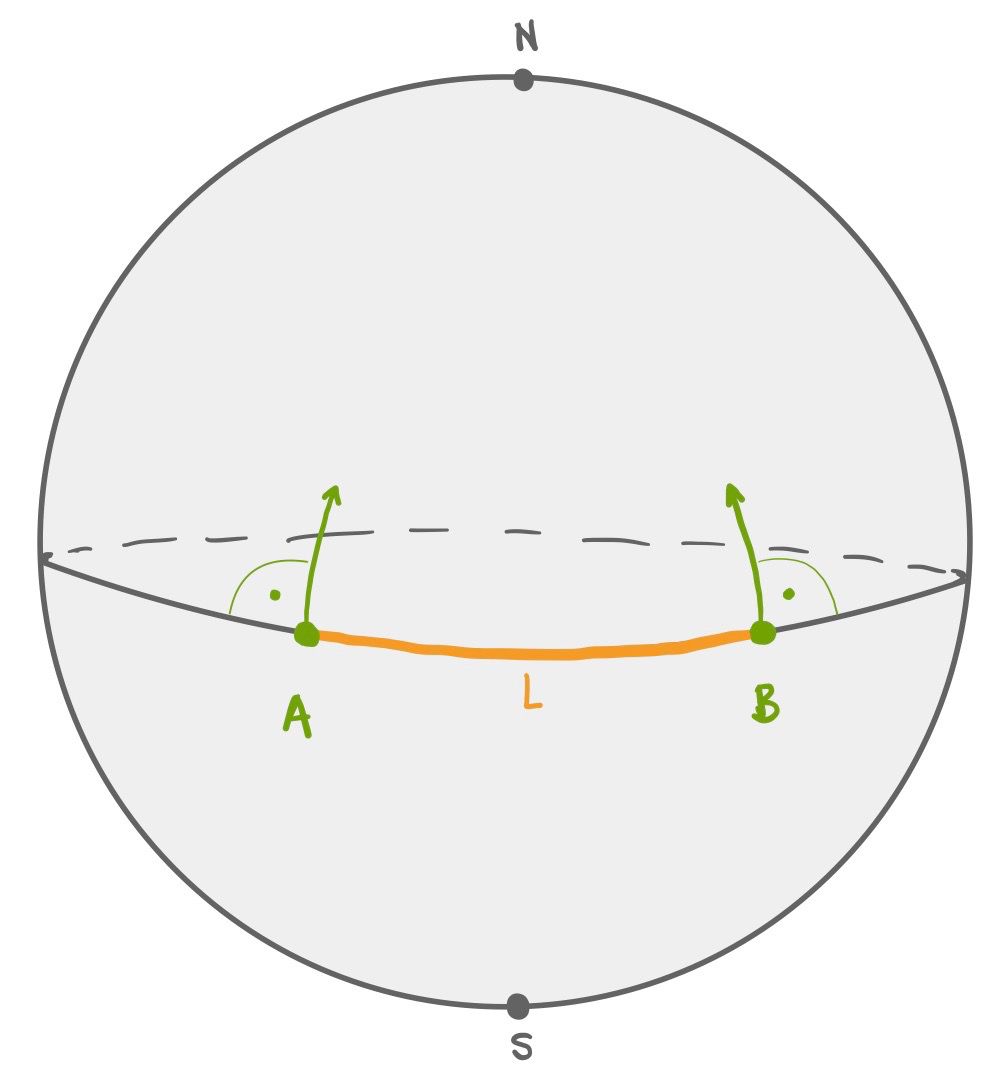
Zatim su uzeli sferu radijusa 1. Počevši od ekvatora, nacrtali su 2 pravca okomita na ekvator. Pravci na početku bili udaljeni za  .
.

Pod kojim će se kutom pravci sječi? (Odgovor upiši u stupnjevima bez mjerne jedinice.) Ako mislite da se pravci nikada ne sijeku upišite 0.
Allice (A) and Bob (B) argued whether two lines pointing in the same direction can meet at some point. First, they try it out on a piece of paper and find that lines pointing in the same direction will never meet or are the same.
Next, they take a sphere of radius 1. Starting on the Equator, they draw two lines perpendicular to the Equator with an initial distance of  .
.

Question: At which angle (in degree) will the two lines meet? Type 0 if you think that the lines will never meet.
