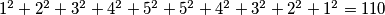Marinada '23

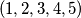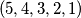[lang = hr] Rješite sustav jednadžbi u pozitivnim realnim brojevima: $$ \begin{aligned} a+h &= m^2\\ e+t &= a^2\\ m+e &= t^2\\ t+a &= h^2\\ h+m &= e^2 \end{aligned} $$ Kao rješenje unesite zbroj kvadrata svih komponenti u svim rješenjima, primjerice ako su rješenja $(1,2,3,4,5)$ i $(5,4,3,2,1)$, kao rješenje upisujete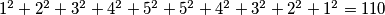 $1^2+2^2+3^2+4^2+5^2+5^2+4^2+3^2+2^2+1^2=110$ [/lang] [lang = en] Solve the system of equations in positive real numbers: $$ \begin{aligned} a+h &= m^2\\ e+t &= a^2\\ m+e &= t^2\\ t+a &= h^2\\ h+m &= e^2 \end{aligned} $$ As a solution, enter the sum of the squares of all components in all solutions, for example if the solutions are 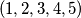$(1,2,3,4,5)$ and $(5,4,3,2,1)$, 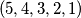you enter $1^2+2^2+3^2+4^2+5^2+5^2+4^2+3^2+2^2+1^2=110$ [/lang]