Vrijeme: 20:40
Zadnji teorem | Last theorem #2
Neka je  najmanji broj takav da kada odaberemo bilo kojih
najmanji broj takav da kada odaberemo bilo kojih  (ne nužno različitih) prirodnih brojeva manjih od
(ne nužno različitih) prirodnih brojeva manjih od 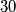 moraju postojati dva čiji je umnožak potpun kvadrat neovisno o odabiru. Koliko iznosi
moraju postojati dva čiji je umnožak potpun kvadrat neovisno o odabiru. Koliko iznosi  ?
?
[/lang = en] Let  najmanji broj takav da kada odaberemo bilo kojih
najmanji broj takav da kada odaberemo bilo kojih  (ne nužno različitih) prirodnih brojeva manjih od
(ne nužno različitih) prirodnih brojeva manjih od 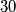 moraju postojati dva čiji je umnožak potpun kvadrat neovisno o odabiru. Koliko iznosi
moraju postojati dva čiji je umnožak potpun kvadrat neovisno o odabiru. Koliko iznosi  ?
?  be the smallest number such that when we choose any
be the smallest number such that when we choose any  (not necessarily distinct) natural numbers smaller than
(not necessarily distinct) natural numbers smaller than 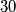 there must be two whose product is a perfect square regardless of the choice. What is
there must be two whose product is a perfect square regardless of the choice. What is  ? [/lang]
? [/lang] 