Vrijeme: 08:30
Prvi teorem | First theorem #5
Nasumično izvlačimo prirodne brojeve manje od 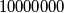 i veće od
i veće od  . Koliko ih moramo izvući da bismo bili sigurni da imamo dva s istim brojem prostih djelitelja (broje se samo različiti prosti faktori, npr.
. Koliko ih moramo izvući da bismo bili sigurni da imamo dva s istim brojem prostih djelitelja (broje se samo različiti prosti faktori, npr.  i
i 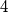 imaju isti broj prostih djelitelja)?
imaju isti broj prostih djelitelja)?
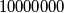 i veće od
i veće od  . Koliko ih moramo izvući da bismo bili sigurni da imamo dva s istim brojem prostih djelitelja (broje se samo različiti prosti faktori, npr.
. Koliko ih moramo izvući da bismo bili sigurni da imamo dva s istim brojem prostih djelitelja (broje se samo različiti prosti faktori, npr.  i
i 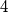 imaju isti broj prostih djelitelja)?
imaju isti broj prostih djelitelja)? We randomly draw natural numbers less than 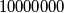 and greater than
and greater than  . How many must we draw to be sure we have two with the same number of prime divisors (only different prime factors are counted, eg
. How many must we draw to be sure we have two with the same number of prime divisors (only different prime factors are counted, eg  and
and 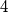 have the same number of prime divisors)?
have the same number of prime divisors)?
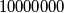 and greater than
and greater than  . How many must we draw to be sure we have two with the same number of prime divisors (only different prime factors are counted, eg
. How many must we draw to be sure we have two with the same number of prime divisors (only different prime factors are counted, eg  and
and 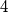 have the same number of prime divisors)?
have the same number of prime divisors)? 