Vrijeme: 15:56
Povratak kutomjera | Return of the protractor #4
Neka je  tetivni četverokut u kome je
tetivni četverokut u kome je 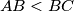 i
i 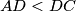 . Neka su
. Neka su  i
i  točke na stranicama
točke na stranicama  i
i  , redom, takve da vrijedi
, redom, takve da vrijedi 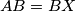 i
i 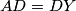 . Neka je
. Neka je  polovište dužine
polovište dužine  . Odredi
. Odredi 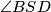 . Rješenje zaokruži na 2 decimale.
. Rješenje zaokruži na 2 decimale.
 tetivni četverokut u kome je
tetivni četverokut u kome je 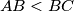 i
i 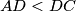 . Neka su
. Neka su  i
i  točke na stranicama
točke na stranicama  i
i  , redom, takve da vrijedi
, redom, takve da vrijedi 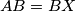 i
i 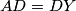 . Neka je
. Neka je  polovište dužine
polovište dužine  . Odredi
. Odredi 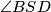 . Rješenje zaokruži na 2 decimale.
. Rješenje zaokruži na 2 decimale. Let  be a cyclic quadrilateral in which
be a cyclic quadrilateral in which 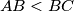 and
and 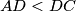 . Let
. Let  and
and  be points on sides
be points on sides  and
and  , respectively, such that
, respectively, such that 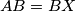 and
and 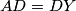 hold. Let
hold. Let  be half the length of
be half the length of  . Determine
. Determine 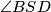 . Round the solution to 2 decimal places.
. Round the solution to 2 decimal places.
 be a cyclic quadrilateral in which
be a cyclic quadrilateral in which 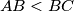 and
and 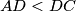 . Let
. Let  and
and  be points on sides
be points on sides  and
and  , respectively, such that
, respectively, such that 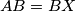 and
and 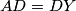 hold. Let
hold. Let  be half the length of
be half the length of  . Determine
. Determine 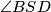 . Round the solution to 2 decimal places.
. Round the solution to 2 decimal places. 