Neka je

najmanja konstanta takva da, za sve realne brojeve
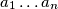
vrijedi:
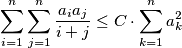
Odredite cijeli broj najbliži broju  .
.
Let

be the smallest constant such that for all real numbers
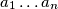
, the following inequality holds:
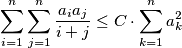
Determine the integer closest to  .
.
[lang=hr]
Neka je $C$ najmanja konstanta takva da, za sve realne brojeve $a_1 \dots a_n$ vrijedi:
\[\sum_{i=1}^n \sum_{j=1}^n \frac{a_ia_j}{i+j} \le C \cdot \sum_{k=1}^n a_k^2\]
Odredite cijeli broj najbliži broju $10000C$.
[/lang]
[lang=en]
Let $C$ be the smallest constant such that for all real numbers $a_1 \dots a_n$, the following inequality holds:
\[\sum_{i=1}^n \sum_{j=1}^n \frac{a_ia_j}{i+j} \le C \cdot \sum_{k=1}^n a_k^2\]
Determine the integer closest to $10000C$.
[/lang]