Školjka Točno
19. prosinca 2022. 13:05 (2 godine, 3 mjeseci)
Let 
be an isosceles triangle with 
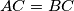, whose incentre is 
. Let 
be a point on the circumcircle of the triangle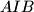 
lying inside the triangle . The lines through parallel to 
and 
meet 
at 
and 
, respectively. The line through parallel to meets and at 
and 
, respectively. Prove that the lines 
and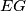 
intersect on the circumcircle of the triangle .
comment
(According to my team leader, last year some of the countries wanted a geometry question that was even easier than this...that explains IMO 2003/4...)
[Note by Darij: This was also Problem 6 of the German pre-TST 2004, written in December 03.]
Edited by Orl.
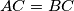be an isosceles triangle with , whose incentre is . Let be a point on the circumcircle of the triangle 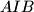lying inside the triangle . The lines through parallel to and meet at and , respectively. The line through parallel to meets and at and , respectively. Prove that the lines and 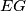intersect on the circumcircle of the triangle . comment
(According to my team leader, last year some of the countries wanted a geometry question that was even easier than this...that explains IMO 2003/4...)
[Note by Darij: This was also Problem 6 of the German pre-TST 2004, written in December 03.]
Edited by Orl.
Upozorenje: Ovaj zadatak još niste riješili!
Kliknite ovdje kako biste prikazali rješenje.
Kliknite ovdje kako biste prikazali rješenje.
Neka je 
presjek 
i . Dokazat ćemo da je na i na 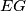. (Motivacija za to je činjenica da su 
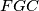i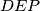 
homotetični pa se 
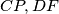i 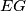sijeku u jednoj točki, pa ako zadatak vrijedi to će morati biti traženi presjek.)
Zbog simetrije dovoljno je da je na . Imamo: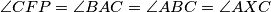 
, odakle je 
tetivan, a kako je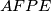 
jednakokračni trapez i leži na toj kružnici. Sličan chase daje i tetivnost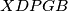 
. Sada, 
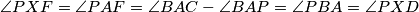što smo i trebali.
P.S. Dosta lagano za jedan G5, nakon nacrtane slike mislim da se i bez ovog argumenta homotetijom može naslutiti gdje će biti ta točka presjeka i od tamo je chase dosta lagan. Ako se usmjere kutevi može se dobiti da zadatak vrijedi i za izvan