There are
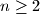
people at a meeting. Show that there exist two people at the meeting who have the same number of friends among the persons at the meeting. (It is assumed that if

is a friend of

then

is a friend of
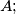
moreover, nobody is his own friend.)
%V0
There are $n\geq 2$ people at a meeting. Show that there exist two people at the meeting who have the same number of friends among the persons at the meeting. (It is assumed that if $A$ is a friend of $B,$ then $B$ is a friend of $A;$ moreover, nobody is his own friend.)