Two concentric circles have radii

and

respectively. Determine the greatest possible number of circles that are tangent to both these circles and mutually nonintersecting. Prove that this number lies between
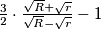
and

%V0
Two concentric circles have radii $R$ and $r$ respectively. Determine the greatest possible number of circles that are tangent to both these circles and mutually nonintersecting. Prove that this number lies between $\frac 32 \cdot \frac{\sqrt R +\sqrt r }{\sqrt R -\sqrt r } -1$ and $\frac{63}{20} \cdot \frac{R+r}{R-r}.$