A polynomial
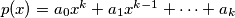
with integer coefficients is said to be divisible by an integer

if
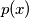
is divisible by m for all integers

. Prove that if
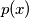
is divisible by

, then

is also divisible by

. Also prove that if
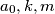
are non-negative integers for which

is divisible by

, there exists a polynomial
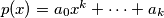
divisible by

%V0
A polynomial $p(x) = a_0x^k + a_1x^{k-1} + \cdots + a_k$ with integer coefficients is said to be divisible by an integer $m$ if $p(x)$ is divisible by m for all integers $x$. Prove that if $p(x)$ is divisible by $m$, then $k!a_0$ is also divisible by $m$. Also prove that if $a_0, k,m$ are non-negative integers for which $k!a_0$ is divisible by $m$, there exists a polynomial $p(x) = a_0x^k+\cdots+ a_k$ divisible by $m.$