We are given a fixed point on the circle of radius  , and going from this point along the circumference in the positive direction on curved distances
, and going from this point along the circumference in the positive direction on curved distances 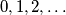 from it we obtain points with abscisas
from it we obtain points with abscisas 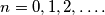 respectively. How many points among them should we take to ensure that some two of them are less than the distance
respectively. How many points among them should we take to ensure that some two of them are less than the distance  apart ?
apart ?
 , and going from this point along the circumference in the positive direction on curved distances
, and going from this point along the circumference in the positive direction on curved distances 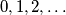 from it we obtain points with abscisas
from it we obtain points with abscisas 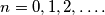 respectively. How many points among them should we take to ensure that some two of them are less than the distance
respectively. How many points among them should we take to ensure that some two of them are less than the distance  apart ?
apart ?  Školjka
Školjka