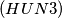
In the plane
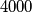
points are given such that each line passes through at most

of these points. Prove that there exist
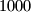
disjoint quadrilaterals in the plane with vertices at these points.
%V0
$(HUN 3)$ In the plane $4000$ points are given such that each line passes through at most $2$ of these points. Prove that there exist $1000$ disjoint quadrilaterals in the plane with vertices at these points.