For which positive integers

do there exist two polynomials

and

with integer coefficients of

variables

such that the following equality is satisfied:
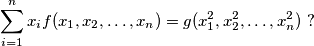
%V0
For which positive integers $n$ do there exist two polynomials $f$ and $g$ with integer coefficients of $n$ variables $x_1, x_2, \ldots , x_n$ such that the following equality is satisfied:
$$\sum_{i=1}^n x_i f(x_1, x_2, \ldots , x_n) = g(x_1^2, x_2^2, \ldots , x_n^2) \ ?$$