Let

be a set of

points in the plane
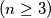
whose coordinates are integers such that any three points from

are vertices of a nondegenerate triangle whose centroid doesnt have both coordinates integers. Determine the maximal

%V0
Let $E$ be a set of $n$ points in the plane $(n \geq 3)$ whose coordinates are integers such that any three points from $E$ are vertices of a nondegenerate triangle whose centroid doesnt have both coordinates integers. Determine the maximal $n.$