Determine the smallest natural number

having the following property: For every integer
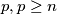
, it is possible to subdivide (partition) a given square into

squares (not necessarily equal).
%V0
Determine the smallest natural number $n$ having the following property: For every integer $p, p \geq n$, it is possible to subdivide (partition) a given square into $p$ squares (not necessarily equal).