(a) Decide whether the fields of the
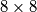
chessboard can be numbered by the numbers
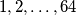
in such a way that the sum of the four numbers in each of its parts of one of the forms
is divisible by four.
(b) Solve the analogous problem for
%V0
(a) Decide whether the fields of the $8 \times 8$ chessboard can be numbered by the numbers $1, 2, \dots , 64$ in such a way that the sum of the four numbers in each of its parts of one of the forms
is divisible by four.
(b) Solve the analogous problem for