Given a set

of
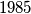
positive integers, none of which has a prime divisor larger than
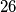
, prove that the set has four distinct elements whose geometric mean is an integer.
%V0
Given a set $M$ of $1985$ positive integers, none of which has a prime divisor larger than $26$, prove that the set has four distinct elements whose geometric mean is an integer.