Given a triangle

, let

and

be points on the side

such that

. If

and

are, respectively, the points of tangency of the incircles of the triangles

and
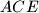
with the line

, then show that
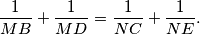
%V0
Given a triangle $ABC$, let $D$ and $E$ be points on the side $BC$ such that $\angle BAD = \angle CAE$. If $M$ and $N$ are, respectively, the points of tangency of the incircles of the triangles $ABD$ and $ACE$ with the line $BC$, then show that
$$\frac{1}{MB}+\frac{1}{MD}= \frac{1}{NC}+\frac{1}{NE}.$$