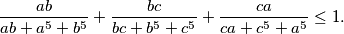Slični zadaci
Let  be an even positive integer. Prove that there exists a positive integer
be an even positive integer. Prove that there exists a positive integer  such that
such that
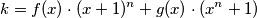
for some polynomials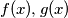 having integer coefficients. If
having integer coefficients. If 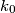 denotes the least such
denotes the least such  determine
determine 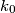 as a function of
as a function of 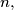 i.e. show that
i.e. show that 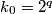 where
where  is the odd integer determined by
is the odd integer determined by 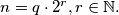
Note: This is variant A6' of the three variants given for this problem.
 be an even positive integer. Prove that there exists a positive integer
be an even positive integer. Prove that there exists a positive integer  such that
such that 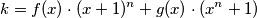
for some polynomials
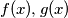 having integer coefficients. If
having integer coefficients. If 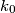 denotes the least such
denotes the least such  determine
determine 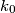 as a function of
as a function of 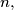 i.e. show that
i.e. show that 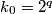 where
where  is the odd integer determined by
is the odd integer determined by 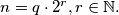
Note: This is variant A6' of the three variants given for this problem.
 Školjka
Školjka  be non-negative reals, not all zero. Show that that
be non-negative reals, not all zero. Show that that 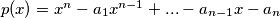 has preceisely 1 positive real root
has preceisely 1 positive real root  .
.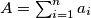 and
and 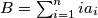 . Show that
. Show that 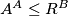 .
. 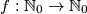 such that for all
such that for all 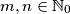 :
: 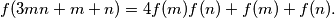
 be the real polynomial function,
be the real polynomial function,  Prove that if
Prove that if 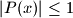 for all
for all  such that
such that 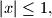 then
then 
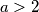 be given, and starting
be given, and starting 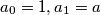 define recursively:
define recursively: 
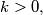 we have:
we have: 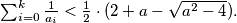
 be real numbers such that for all integers
be real numbers such that for all integers 
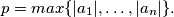 Prove that
Prove that  and that
and that 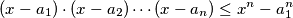 for all
for all 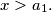
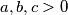 such that
such that 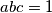 . Prove that
. Prove that