Let

be an interior point of acute triangle

. Let

lie on

with

perpendicular to

. Define

on

and

on

similarly. Prove that

is the circumcenter of

if and only if the perimeter of
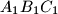
is not less than any one of the perimeters of
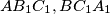
, and
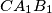
.
%V0
Let $O$ be an interior point of acute triangle $ABC$. Let $A_1$ lie on $BC$ with $OA_1$ perpendicular to $BC$. Define $B_1$ on $CA$ and $C_1$ on $AB$ similarly. Prove that $O$ is the circumcenter of $ABC$ if and only if the perimeter of $A_1B_1C_1$ is not less than any one of the perimeters of $AB_1C_1, BC_1A_1$, and $CA_1B_1$.