Find all functions
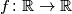
satisfying the equation
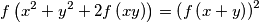
for all
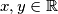
.
%V0
Find all functions $f\colon\mathbb{R} \rightarrow\mathbb{R}$ satisfying the equation $$f\left(x^2 + y^2 + 2f\left(xy\right)\right) = \left(f\left(x + y\right)\right)^2$$ for all $x,y\in \mathbb{R}$.