Školjka

%V0 Let $ABCD$ be a convex quadrilateral and let $P$ and $Q$ be points in $ABCD$ such that $PQDA$ and $QPBC$ are cyclic quadrilaterals. Suppose that there exists a point $E$ on the line segment $PQ$ such that $\angle PAE = \angle QDE$ and $\angle PBE = \angle QCE$. Show that the quadrilateral $ABCD$ is cyclic. Proposed by John Cuya, Peru

%V0 Let $ABC$ be a trapezoid with parallel sides $AB > CD$. Points $K$ and $L$ lie on the line segments $AB$ and $CD$, respectively, so that $\frac {AK}{KB} = \frac {DL}{LC}$. Suppose that there are points $P$ and $Q$ on the line segment $KL$ satisfying $\angle{APB} = \angle{BCD}$ and $\angle{CQD} = \angle{ABC}$. Prove that the points $P$, $Q$, $B$ and $C$ are concylic.

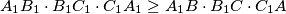
%V0 Let $ABC$ be an equilateral triangle and let $P$ be a point in its interior. Let the lines $AP$, $BP$, $CP$ meet the sides $BC$, $CA$, $AB$ at the points $A_1$, $B_1$, $C_1$, respectively. Prove that $A_1B_1 \cdot B_1C_1 \cdot C_1A_1 \ge A_1B \cdot B_1C \cdot C_1A$.

%V0 Let $O$ be the circumcenter and $H$ the orthocenter of an acute-angled triangle $ABC$ such that $BC>CA$. Let $F$ be the foot of the altitude $CH$ of triangle $ABC$. The perpendicular to the line $OF$ at the point $F$ intersects the line $AC$ at $P$. Prove that $\measuredangle FHP=\measuredangle BAC$.

%V0 Let $A, B$ and $C$ be non-collinear points. Prove that there is a unique point $X$ in the plane of $ABC$ such that $$XA^2 + XB^2 + AB^2 = XB^2 + XC^2 + BC^2 = XC^2 + XA^2 + CA^2.$$

%V0 $ABCD$ is a quadrilateral with $BC$ parallel to $AD$. $M$ is the midpoint of $CD$, $P$ is the midpoint of $MA$ and $Q$ is the midpoint of $MB$. The lines $DP$ and $CQ$ meet at $N$. Prove that $N$ is inside the quadrilateral $ABCD$.