Let

,

,

be real numbers such that for every two of the equations
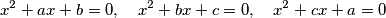
there is exactly one real number satisfying both of them. Determine all possible values of
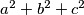
.
%V0
Let $a$, $b$, $c$ be real numbers such that for every two of the equations $$x^2+ax+b=0, \quad x^2+bx+c=0, \quad x^2+cx+a=0$$ there is exactly one real number satisfying both of them. Determine all possible values of $a^2+b^2+c^2$.