We call a positive integer

amazing if there exist positive integers

such that the equality
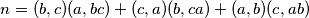
holds. Prove that there exist
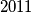
consecutive positive integers which are amazing.
Note. By
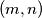
we denote the greatest common divisor of positive integers

and

.
%V0
We call a positive integer $n$ amazing if there exist positive integers $a, b, c$ such that the equality $$n = (b, c)(a, bc) + (c, a)(b, ca) + (a, b)(c, ab)$$ holds. Prove that there exist $2011$ consecutive positive integers which are amazing.
Note. By $(m, n)$ we denote the greatest common divisor of positive integers $m$ and $n$.