Let  be a positive integer. Consider words of length
be a positive integer. Consider words of length  composed of letters from the set
composed of letters from the set 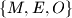 . Let
. Let  be the number of such words containing an even number (possibly 0) of blocks
be the number of such words containing an even number (possibly 0) of blocks 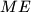 and an even number (possibly 0) blocks of
and an even number (possibly 0) blocks of 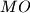 . Similarly let
. Similarly let  the number of such words containing an odd number of blocks
the number of such words containing an odd number of blocks 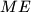 and an odd number of blocks
and an odd number of blocks 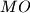 . Prove that
. Prove that 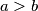 .
.
 be a positive integer. Consider words of length
be a positive integer. Consider words of length  composed of letters from the set
composed of letters from the set 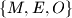 . Let
. Let  be the number of such words containing an even number (possibly 0) of blocks
be the number of such words containing an even number (possibly 0) of blocks 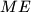 and an even number (possibly 0) blocks of
and an even number (possibly 0) blocks of 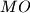 . Similarly let
. Similarly let  the number of such words containing an odd number of blocks
the number of such words containing an odd number of blocks 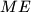 and an odd number of blocks
and an odd number of blocks 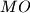 . Prove that
. Prove that 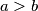 .
.  Školjka
Školjka