In an acute triangle

the points
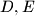
and

are the feet of the altitudes through
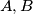
and

respectively. The incenters of the triangles
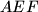
and
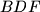
are
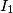
and
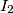
respectively; the circumcenters of the triangles
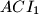
and

are
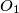
and
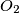
respectively. Prove that

and
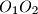
are parallel.
%V0
In an acute triangle $ABC$ the points $D,E$ and $F$ are the feet of the altitudes through $A,B$ and $C$ respectively. The incenters of the triangles $AEF$ and $BDF$ are $I_1$ and $I_2$ respectively; the circumcenters of the triangles $ACI_1$ and $BCI_2$ are $O_1$ and $O_2$ respectively. Prove that $I_1I_2$ and $O_1O_2$ are parallel.