Consider  lines in the plane such that no two lines are parallel and no three have a common point. These lines divide the plane into polygonal regions; let
lines in the plane such that no two lines are parallel and no three have a common point. These lines divide the plane into polygonal regions; let  be the set of regions having finite area. Prove that it is possible to colour
be the set of regions having finite area. Prove that it is possible to colour 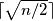 of the lines blue in such a way that no region in
of the lines blue in such a way that no region in  has a completely blue boundary. (For a real number
has a completely blue boundary. (For a real number  ,
, 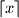 denotes the least integer which is not smaller than
denotes the least integer which is not smaller than  .)
.)
(Austria)
 Školjka
Školjka