Let  be the incentre of a non-equilateral triangle
be the incentre of a non-equilateral triangle  ,
, 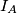 be the
be the  -excentre,
-excentre, 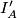 be the reflection of
be the reflection of 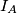 in
in  , and
, and 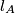 be the reflection of line
be the reflection of line 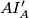 in
in 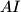 . Define points
. Define points 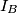 ,
, 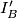 and line
and line 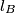 analogously. Let
analogously. Let  be the intersection point of
be the intersection point of 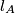 and
and 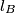 .
.
(a) Prove that  lies on line
lies on line 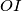 where
where  is the circumcentre of triangle
is the circumcentre of triangle  .
.
(b) Let one of the tangents from  to the incircle of triangle
to the incircle of triangle  meet the circumcircle at points
meet the circumcircle at points  and
and  . Show that
. Show that 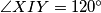 .
.
 Školjka
Školjka