Slični zadaci
Consider a circle with center  and radius
and radius 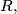 and let
and let  and
and  be two points in the plane of this circle.
be two points in the plane of this circle.
a.) Draw a chord of the circle such that
of the circle such that  is parallel to
is parallel to 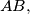 and the point of the intersection
and the point of the intersection  of the lines
of the lines  and
and  lies on the circle.
lies on the circle.
b.) Show that generally, one gets two possible points (
(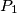 and
and 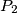 ) satisfying the condition of the above problem, and compute the distance between these two points, if the lengths
) satisfying the condition of the above problem, and compute the distance between these two points, if the lengths 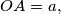
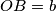 and
and 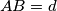 are given.
are given.
 and radius
and radius 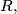 and let
and let  and
and  be two points in the plane of this circle.
be two points in the plane of this circle. a.) Draw a chord
 of the circle such that
of the circle such that  is parallel to
is parallel to 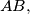 and the point of the intersection
and the point of the intersection  of the lines
of the lines  and
and  lies on the circle.
lies on the circle. b.) Show that generally, one gets two possible points
 (
(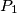 and
and 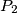 ) satisfying the condition of the above problem, and compute the distance between these two points, if the lengths
) satisfying the condition of the above problem, and compute the distance between these two points, if the lengths 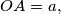
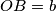 and
and 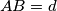 are given.
are given.  Školjka
Školjka  and
and  respectively. Determine the greatest possible number of circles that are tangent to both these circles and mutually nonintersecting. Prove that this number lies between
respectively. Determine the greatest possible number of circles that are tangent to both these circles and mutually nonintersecting. Prove that this number lies between 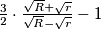 and
and 
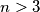 points in the plane such that no three of the points are collinear. Does there exist a circle passing through (at least)
points in the plane such that no three of the points are collinear. Does there exist a circle passing through (at least)  of the given points and not containing any other of the
of the given points and not containing any other of the  points in its interior ?
points in its interior ? 


 on a circle such that
on a circle such that  is a diameter and
is a diameter and  and
and 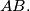
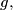 and given a circle
and given a circle 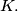 Construct a circle passing through the point
Construct a circle passing through the point  and the line
and the line 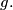
 in the plane any two points of which are connectable by a semicircle lying in
in the plane any two points of which are connectable by a semicircle lying in