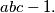In a mathematical contest, three problems,  were posed. Among the participants ther were 25 students who solved at least one problem each. Of all the contestants who did not solve problem
were posed. Among the participants ther were 25 students who solved at least one problem each. Of all the contestants who did not solve problem  , the number who solved
, the number who solved  was twice the number who solved
was twice the number who solved  . The number of students who solved only problem
. The number of students who solved only problem  was one more than the number of students who solved
was one more than the number of students who solved  and at least one other problem. Of all students who solved just one problem, half did not solve problem
and at least one other problem. Of all students who solved just one problem, half did not solve problem  . How many students solved only problem
. How many students solved only problem  ?
?
 were posed. Among the participants ther were 25 students who solved at least one problem each. Of all the contestants who did not solve problem
were posed. Among the participants ther were 25 students who solved at least one problem each. Of all the contestants who did not solve problem  , the number who solved
, the number who solved  was twice the number who solved
was twice the number who solved  . The number of students who solved only problem
. The number of students who solved only problem  was one more than the number of students who solved
was one more than the number of students who solved  and at least one other problem. Of all students who solved just one problem, half did not solve problem
and at least one other problem. Of all students who solved just one problem, half did not solve problem  . How many students solved only problem
. How many students solved only problem  ?
?  Školjka
Školjka  and
and  are natural numbers so that
are natural numbers so that 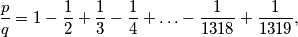 prove that
prove that 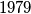 .
. 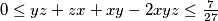 , where
, where  and
and  are non-negative real numbers satisfying
are non-negative real numbers satisfying 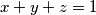 .
.  be any positive integer not equal to
be any positive integer not equal to 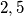 or
or 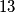 . Show that one can find distinct
. Show that one can find distinct 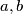 in the set
in the set 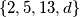 such that
such that 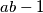 is not a perfect square.
is not a perfect square. 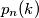 be the number of permutations of the set
be the number of permutations of the set 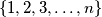 which have exactly
which have exactly  fixed points. Prove that
fixed points. Prove that 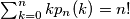 .(IMO Problem 1)
.(IMO Problem 1) be a set of
be a set of  elements. We denote the number of all permutations of
elements. We denote the number of all permutations of 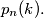 Prove:
Prove: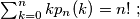
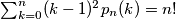
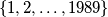 can be expressed as the disjoint union of subsets
can be expressed as the disjoint union of subsets 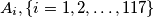 such that
such that 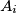 contains 17 elements
contains 17 elements 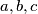 with
with 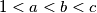 such that
such that 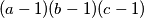 is a divisor of
is a divisor of