Slični zadaci
Consider a plane  and three non-collinear points
and three non-collinear points  on the same side of
on the same side of  ; suppose the plane determined by these three points is not parallel to
; suppose the plane determined by these three points is not parallel to  . In plane
. In plane  take three arbitrary points
take three arbitrary points 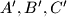 . Let
. Let 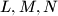 be the midpoints of segments
be the midpoints of segments 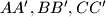 ; Let
; Let  be the centroid of the triangle
be the centroid of the triangle 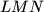 . (We will not consider positions of the points
. (We will not consider positions of the points  such that the points
such that the points 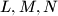 do not form a triangle.) What is the locus of point
do not form a triangle.) What is the locus of point  as
as  range independently over the plane
range independently over the plane  ?
?
 and three non-collinear points
and three non-collinear points  on the same side of
on the same side of  ; suppose the plane determined by these three points is not parallel to
; suppose the plane determined by these three points is not parallel to  . In plane
. In plane  take three arbitrary points
take three arbitrary points 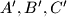 . Let
. Let 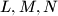 be the midpoints of segments
be the midpoints of segments 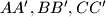 ; Let
; Let  be the centroid of the triangle
be the centroid of the triangle 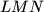 . (We will not consider positions of the points
. (We will not consider positions of the points  such that the points
such that the points 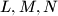 do not form a triangle.) What is the locus of point
do not form a triangle.) What is the locus of point  as
as  range independently over the plane
range independently over the plane  ?
? Let  be a triangle, and let
be a triangle, and let  ,
,  ,
,  be three points in the interiors of the sides
be three points in the interiors of the sides  ,
,  ,
,  of this triangle. Prove that the area of at least one of the three triangles
of this triangle. Prove that the area of at least one of the three triangles 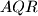 ,
, 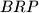 ,
, 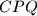 is less than or equal to one quarter of the area of triangle
is less than or equal to one quarter of the area of triangle  .
.
Alternative formulation: Let be a triangle, and let
be a triangle, and let  ,
,  ,
,  be three points on the segments
be three points on the segments  ,
,  ,
,  , respectively. Prove that
, respectively. Prove that
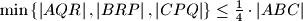 ,
,
where the abbreviation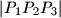 denotes the (non-directed) area of an arbitrary triangle
denotes the (non-directed) area of an arbitrary triangle 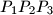 .
.
 be a triangle, and let
be a triangle, and let  ,
,  ,
,  be three points in the interiors of the sides
be three points in the interiors of the sides  ,
,  ,
,  of this triangle. Prove that the area of at least one of the three triangles
of this triangle. Prove that the area of at least one of the three triangles 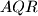 ,
, 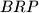 ,
, 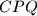 is less than or equal to one quarter of the area of triangle
is less than or equal to one quarter of the area of triangle  .
. Alternative formulation: Let
 be a triangle, and let
be a triangle, and let  ,
,  ,
,  be three points on the segments
be three points on the segments  ,
,  ,
,  , respectively. Prove that
, respectively. Prove that 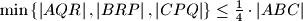 ,
, where the abbreviation
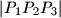 denotes the (non-directed) area of an arbitrary triangle
denotes the (non-directed) area of an arbitrary triangle 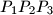 .
.  Školjka
Školjka  within the triangle. Lines
within the triangle. Lines 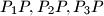 intersect the opposite sides in points
intersect the opposite sides in points 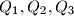 respectively. Prove that, of the numbers
respectively. Prove that, of the numbers 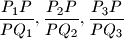
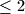 and at least one is
and at least one is 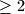
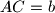 ,
, 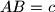 and
and 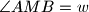 , where
, where  is the midpoint of the segment
is the midpoint of the segment 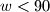 . Prove that a solution exists if and only if
. Prove that a solution exists if and only if 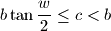 In what case does the equality hold?
In what case does the equality hold? 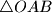 with acute angle
with acute angle 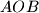 . Thorugh a point
. Thorugh a point 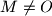 perpendiculars are drawn to
perpendiculars are drawn to 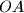 and
and 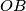 , the feet of which are
, the feet of which are 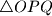 is
is  . What is the locus of
. What is the locus of  be the lengths of the sides of a triangle, and
be the lengths of the sides of a triangle, and 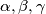 respectively, the angles opposite these sides. Prove that if
respectively, the angles opposite these sides. Prove that if 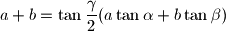 the triangle is isosceles.
the triangle is isosceles.