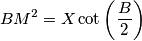Slični zadaci
Let  be an acute-angled triangle. The lines
be an acute-angled triangle. The lines  ,
, 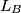 and
and 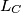 are constructed through the vertices
are constructed through the vertices  ,
,  and
and  respectively according the following prescription: Let
respectively according the following prescription: Let  be the foot of the altitude drawn from the vertex
be the foot of the altitude drawn from the vertex  to the side
to the side  ; let
; let 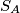 be the circle with diameter
be the circle with diameter  ; let
; let 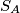 meet the sides
meet the sides  and
and  at
at  and
and  respectively, where
respectively, where  and
and  are distinct from
are distinct from  ; then let
; then let  be the line through
be the line through  perpendicular to
perpendicular to  . The lines
. The lines 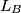 and
and 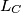 are constructed similarly. Prove that the lines
are constructed similarly. Prove that the lines  ,
, 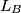 and
and 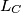 are concurrent.
are concurrent.
 be an acute-angled triangle. The lines
be an acute-angled triangle. The lines  ,
, 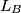 and
and 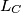 are constructed through the vertices
are constructed through the vertices  ,
,  and
and  respectively according the following prescription: Let
respectively according the following prescription: Let  be the foot of the altitude drawn from the vertex
be the foot of the altitude drawn from the vertex  to the side
to the side  ; let
; let 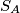 be the circle with diameter
be the circle with diameter  ; let
; let 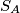 meet the sides
meet the sides  and
and  at
at  and
and  respectively, where
respectively, where  and
and  are distinct from
are distinct from  ; then let
; then let  be the line through
be the line through  perpendicular to
perpendicular to  . The lines
. The lines 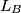 and
and 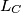 are constructed similarly. Prove that the lines
are constructed similarly. Prove that the lines  ,
, 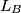 and
and 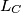 are concurrent.
are concurrent.  Školjka
Školjka  Find the radius of the circle circumscribed about the isosceles triangle whose sides are the solutions of the equation
Find the radius of the circle circumscribed about the isosceles triangle whose sides are the solutions of the equation 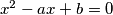 .
. 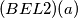 Find the equations of regular hyperbolas passing through the points
Find the equations of regular hyperbolas passing through the points  and
and 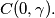
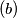 Prove that all such hyperbolas pass through the orthocenter
Prove that all such hyperbolas pass through the orthocenter 
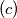 Find the locus of the centers of these hyperbolas.
Find the locus of the centers of these hyperbolas. Check whether this locus coincides with the nine-point circle of the triangle
Check whether this locus coincides with the nine-point circle of the triangle 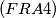 A right-angled triangle
A right-angled triangle 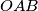 has its right angle at the point
has its right angle at the point 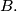 An arbitrary circle with center on the line
An arbitrary circle with center on the line 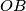 is tangent to the line
is tangent to the line 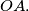 Let
Let 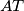 be the tangent to the circle different from
be the tangent to the circle different from 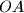 (
( is the point of tangency). Prove that the median from
is the point of tangency). Prove that the median from 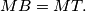
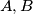 and
and 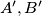 and
and 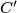 respectively. Prove that the area of triangle
respectively. Prove that the area of triangle 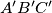 is greater than or equal to the area of triangle
is greater than or equal to the area of triangle  be the centre of the inscribed circle of a triangle
be the centre of the inscribed circle of a triangle 
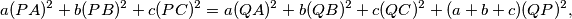
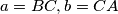 and
and 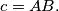
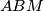 and
and  are equal. Prove that
are equal. Prove that