We have

for
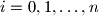
and
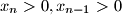
. If
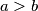
, and
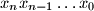
represents the number

base

and

base

, whilst
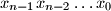
represents the number
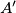
base

and
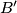
base

, prove that
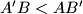
.
%V0
We have $0\le x_i<b$ for $i=0,1,\ldots,n$ and $x_n>0,x_{n-1}>0$. If $a>b$, and $x_nx_{n-1}\ldots x_0$ represents the number $A$ base $a$ and $B$ base $b$, whilst $x_{n-1}x_{n-2}\ldots x_0$ represents the number $A'$ base $a$ and $B'$ base $b$, prove that $A'B<AB'$.