Let

and
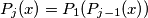
for j
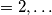
Prove that for any positive integer n the roots of the equation
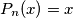
are all real and distinct.
%V0
Let $P_{1}(x)=x^{2}-2$ and $P_{j}(x)=P_{1}(P_{j-1}(x))$ for j$=2,\ldots$ Prove that for any positive integer n the roots of the equation $P_{n}(x)=x$ are all real and distinct.