Slični zadaci
Let  be a given number greater than 2. We consider the set
be a given number greater than 2. We consider the set  of all the integers of the form
of all the integers of the form 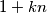 with
with 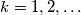 A number
A number  from
from  is called indecomposable in
is called indecomposable in  if there are not two numbers
if there are not two numbers  and
and  from
from  so that
so that 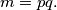 Prove that there exist a number
Prove that there exist a number 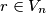 that can be expressed as the product of elements indecomposable in
that can be expressed as the product of elements indecomposable in  in more than one way. (Expressions which differ only in order of the elements of
in more than one way. (Expressions which differ only in order of the elements of  will be considered the same.)
will be considered the same.)
 be a given number greater than 2. We consider the set
be a given number greater than 2. We consider the set  of all the integers of the form
of all the integers of the form 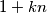 with
with 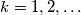 A number
A number  from
from  is called indecomposable in
is called indecomposable in  if there are not two numbers
if there are not two numbers  and
and  from
from  so that
so that 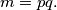 Prove that there exist a number
Prove that there exist a number 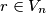 that can be expressed as the product of elements indecomposable in
that can be expressed as the product of elements indecomposable in  in more than one way. (Expressions which differ only in order of the elements of
in more than one way. (Expressions which differ only in order of the elements of  will be considered the same.)
will be considered the same.) Given an initial integer 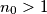 , two players,
, two players,  and
and  , choose integers
, choose integers 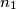 ,
, 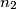 ,
, 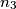 ,
,  alternately according to the following rules :
alternately according to the following rules :
I.) Knowing ,
,  chooses any integer
chooses any integer 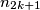 such that
such that
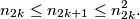
II.) Knowing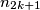 ,
,  chooses any integer
chooses any integer 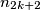 such that
such that
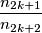
is a prime raised to a positive integer power.
Player wins the game by choosing the number 1990; player
wins the game by choosing the number 1990; player  wins by choosing the number 1. For which
wins by choosing the number 1. For which  does :
does :
a.) have a winning strategy?
have a winning strategy?
b.) have a winning strategy?
have a winning strategy?
c.) Neither player have a winning strategy?
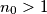 , two players,
, two players,  and
and  , choose integers
, choose integers 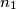 ,
, 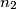 ,
, 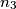 ,
,  alternately according to the following rules :
alternately according to the following rules : I.) Knowing
 ,
,  chooses any integer
chooses any integer 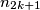 such that
such that 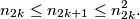
II.) Knowing
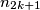 ,
,  chooses any integer
chooses any integer 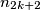 such that
such that 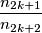
is a prime raised to a positive integer power.
Player
 wins the game by choosing the number 1990; player
wins the game by choosing the number 1990; player  wins by choosing the number 1. For which
wins by choosing the number 1. For which  does :
does : a.)
 have a winning strategy?
have a winning strategy? b.)
 have a winning strategy?
have a winning strategy? c.) Neither player have a winning strategy?
 Školjka
Školjka 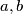 be two natural numbers. When we divide
be two natural numbers. When we divide  by
by 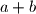 , we the the remainder
, we the the remainder  and the quotient
and the quotient 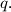 Determine all pairs
Determine all pairs 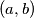 for which
for which 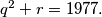

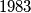 distinct positive integers, all less than or equal to
distinct positive integers, all less than or equal to 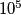 , no three of which are consecutive terms of an arithmetic progression?
, no three of which are consecutive terms of an arithmetic progression? 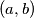 of positive integers that satisfy the equation:
of positive integers that satisfy the equation: 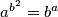 .
.