Slični zadaci
An isosceles trapezoid with bases  and
and  and altitude
and altitude  is given.
is given.
a) On the axis of symmetry of this trapezoid, find all points such that both legs of the trapezoid subtend right angles at
such that both legs of the trapezoid subtend right angles at  ;
;
b) Calculate the distance of from either base;
from either base;
c) Determine under what conditions such points actually exist. Discuss various cases that might arise.
actually exist. Discuss various cases that might arise.
 and
and  and altitude
and altitude  is given.
is given. a) On the axis of symmetry of this trapezoid, find all points
 such that both legs of the trapezoid subtend right angles at
such that both legs of the trapezoid subtend right angles at  ;
; b) Calculate the distance of
 from either base;
from either base; c) Determine under what conditions such points
 actually exist. Discuss various cases that might arise.
actually exist. Discuss various cases that might arise. Supppose five points in a plane are situated so that no two of the straight lines joining them are parallel, perpendicular, or coincident. From each point perpendiculars are drawn to all the lines joining the other four points. Determine the maxium number of intersections that these perpendiculars can have.
 Školjka
Školjka  and
and 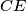 of the regular hexagon
of the regular hexagon 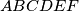 are divided by inner points
are divided by inner points  and
and  respectively, so that
respectively, so that 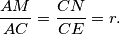 Determine
Determine  if
if  and
and  if
if 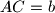 ,
, 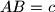 and
and 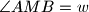 , where
, where  and
and 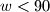 . Prove that a solution exists if and only if
. Prove that a solution exists if and only if 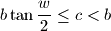 In what case does the equality hold?
In what case does the equality hold?  and segment
and segment  -gon
-gon 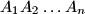 , all of whose interior angles are equal, the lengths of consecutive sides satisfy the relation
, all of whose interior angles are equal, the lengths of consecutive sides satisfy the relation 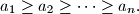
 .
. 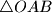 with acute angle
with acute angle 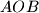 . Thorugh a point
. Thorugh a point 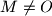 perpendiculars are drawn to
perpendiculars are drawn to 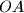 and
and 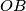 , the feet of which are
, the feet of which are  respectively. The point of intersection of the altitudes of
respectively. The point of intersection of the altitudes of 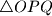 is
is  . What is the locus of
. What is the locus of  ;
;