Let
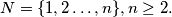
A collection
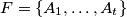
of subsets
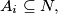

is said to be separating, if for every pair

there is a set
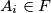
so that
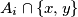
contains just one element.

is said to be covering, if every element of

is contained in at least one set
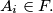
What is the smallest value

of
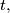
so there is a set
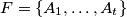
which is simultaneously separating and covering?
%V0
Let $N = \{1,2 \ldots, n\}, n \geq 2.$ A collection $F = \{A_1, \ldots, A_t\}$ of subsets $A_i \subseteq N,$ $i = 1, \ldots, t,$ is said to be separating, if for every pair $\{x,y\} \subseteq N,$ there is a set $A_i \in F$ so that $A_i \cap \{x,y\}$ contains just one element. $F$ is said to be covering, if every element of $N$ is contained in at least one set $A_i \in F.$ What is the smallest value $f(n)$ of $t,$ so there is a set $F = \{A_1, \ldots, A_t\}$ which is simultaneously separating and covering?