Find the least natural number

such that, if the set
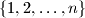
is arbitrarily divided into two non-intersecting subsets, then one of the subsets contains 3 distinct numbers such that the product of two of them equals the third.
%V0
Find the least natural number $n$ such that, if the set $\{1,2, \ldots, n\}$ is arbitrarily divided into two non-intersecting subsets, then one of the subsets contains 3 distinct numbers such that the product of two of them equals the third.