Slični zadaci
Let  be a triangle. Prove that there exists a point
be a triangle. Prove that there exists a point  on the side
on the side  of the triangle
of the triangle  , such that
, such that  is the geometric mean of
is the geometric mean of  and
and 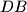 , iff the triangle
, iff the triangle  satisfies the inequality
satisfies the inequality 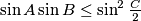 .
.
CommentAlternative formulation, from IMO ShortList 1974, Finland 2: We consider a triangle . Prove that:
. Prove that: 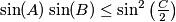 is a necessary and sufficient condition for the existence of a point
is a necessary and sufficient condition for the existence of a point  on the segment
on the segment  so that
so that  is the geometrical mean of
is the geometrical mean of  and
and  .
.
 be a triangle. Prove that there exists a point
be a triangle. Prove that there exists a point  on the side
on the side  of the triangle
of the triangle  , such that
, such that  is the geometric mean of
is the geometric mean of  and
and 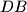 , iff the triangle
, iff the triangle  satisfies the inequality
satisfies the inequality 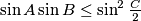 .
. CommentAlternative formulation, from IMO ShortList 1974, Finland 2: We consider a triangle
 . Prove that:
. Prove that: 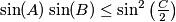 is a necessary and sufficient condition for the existence of a point
is a necessary and sufficient condition for the existence of a point  on the segment
on the segment  so that
so that  is the geometrical mean of
is the geometrical mean of  and
and  .
.  Školjka
Školjka  be any line in the plane of the triangle
be any line in the plane of the triangle  ,
,  ,
,  the lengths of the perpendiculars to
the lengths of the perpendiculars to  ,
,  ,
,  respectively. Prove the inequality
respectively. Prove the inequality 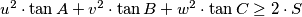 , where
, where  is the area of the triangle
is the area of the triangle  , of length
, of length  , is divided into
, is divided into  equal parts (
equal parts (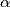 be the acute angel subtending, from
be the acute angel subtending, from  be the length of the altitude to the hypotenuse fo the triangle. Prove that:
be the length of the altitude to the hypotenuse fo the triangle. Prove that: 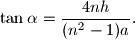
 and
and 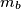 of a triangle are orthogonal. Prove that:
of a triangle are orthogonal. Prove that: 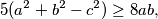 is valid, where
is valid, where 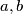 and
and  are side length of the given triangle.
are side length of the given triangle.  a point inside it. Let
a point inside it. Let 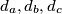 be the distances from
be the distances from  ;
;  the lengths of the sides respectively, and
the lengths of the sides respectively, and 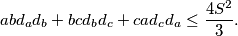
 , then
, then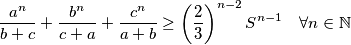
 let
let  be altitudes and
be altitudes and 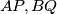 internal bisectors. Denote by
internal bisectors. Denote by  and
and  the incenter and the circumcentre of the triangle, respectively. Prove that the points
the incenter and the circumcentre of the triangle, respectively. Prove that the points 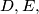 and
and 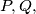 and
and