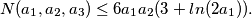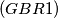
The polynomial

, where
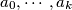
are integers, is said to be divisible by an integer

if

is a multiple of

for every integral value of

. Show that if

is divisible by

, then
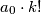
is a multiple of

. Also prove that if
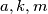
are positive integers such that
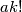
is a multiple of

, then a polynomial

with leading term
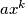
can be found that is divisible by

%V0
$(GBR 1)$ The polynomial $P(x) = a_0x^k + a_1x^{k-1} + \cdots + a_k$, where $a_0,\cdots, a_k$ are integers, is said to be divisible by an integer $m$ if $P(x)$ is a multiple of $m$ for every integral value of $x$. Show that if $P(x)$ is divisible by $m$, then $a_0 \cdot k!$ is a multiple of $m$. Also prove that if $a, k,m$ are positive integers such that $ak!$ is a multiple of $m$, then a polynomial $P(x)$ with leading term $ax^k$can be found that is divisible by $m.$
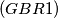 The polynomial
The polynomial  , where
, where 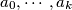 are integers, is said to be divisible by an integer
are integers, is said to be divisible by an integer  if
if  is a multiple of
is a multiple of  for every integral value of
for every integral value of  . Show that if
. Show that if  is divisible by
is divisible by  , then
, then 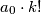 is a multiple of
is a multiple of  . Also prove that if
. Also prove that if 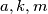 are positive integers such that
are positive integers such that 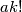 is a multiple of
is a multiple of  , then a polynomial
, then a polynomial  with leading term
with leading term 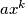 can be found that is divisible by
can be found that is divisible by 
 Školjka
Školjka  there exists uniquely determined integers
there exists uniquely determined integers 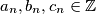 such
such ![\left(1 + 4 \cdot \sqrt[3]{2} - 4 \cdot \sqrt[3]{4} \right)^n = a_n + b_n \cdot \sqrt[3]{2} + c_n \cdot \sqrt[3]{4}.](/media/m/9/7/8/97803f4eeaf0e47fd00e9b1ea8ad4f40.png)
 implies
implies 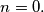
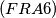 Consider the integer
Consider the integer  , where
, where 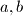 , and
, and  are positive integers and
are positive integers and 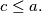 Prove that the set
Prove that the set  of integers that are between
of integers that are between  and
and  and relatively prime to
and relatively prime to  ) can be partitioned into
) can be partitioned into  subsets, each of which consists of
subsets, each of which consists of  elements. What can be said about the rational number
elements. What can be said about the rational number 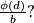
 Let
Let  be positive integers such that
be positive integers such that  and
and 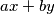 is
is  . If
. If 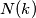 is the largest number not expressible in the form
is the largest number not expressible in the form  ways, find
ways, find 
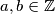 which are not perfect squares. Prove that if
which are not perfect squares. Prove that if 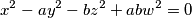 has a nontrivial solution in integers, then so does
has a nontrivial solution in integers, then so does 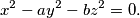
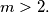 Find the smallest positive integer
Find the smallest positive integer 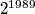 divides
divides 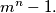
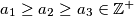 be given and let N
be given and let N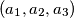 be the number of solutions
be the number of solutions 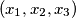 of the equation
of the equation 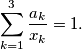
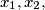 and
and  are positive integers. Prove that
are positive integers. Prove that