Slični zadaci
Let  be a triangle, and let the angle bisectors of its angles
be a triangle, and let the angle bisectors of its angles 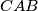 and
and  meet the sides
meet the sides  and
and  at the points
at the points  and
and  , respectively. The lines
, respectively. The lines  and
and 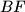 meet the line through the point
meet the line through the point  parallel to
parallel to  at the points
at the points  and
and  respectively, and we have
respectively, and we have 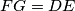 . Prove that
. Prove that 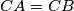 .
.
Original formulation:
Let be a triangle and
be a triangle and  the line through
the line through  parallel to the side
parallel to the side 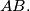 Let the internal bisector of the angle at
Let the internal bisector of the angle at  meet the side
meet the side  at
at  and the line
and the line  at
at  and let the internal bisector of the angle at
and let the internal bisector of the angle at  meet the side
meet the side  at
at  and the line
and the line  at
at  If
If 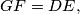 prove that
prove that 
 be a triangle, and let the angle bisectors of its angles
be a triangle, and let the angle bisectors of its angles 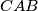 and
and  meet the sides
meet the sides  and
and  at the points
at the points  and
and  , respectively. The lines
, respectively. The lines  and
and 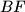 meet the line through the point
meet the line through the point  parallel to
parallel to  at the points
at the points  and
and  respectively, and we have
respectively, and we have 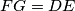 . Prove that
. Prove that 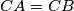 .
. Original formulation:
Let
 be a triangle and
be a triangle and  the line through
the line through  parallel to the side
parallel to the side 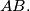 Let the internal bisector of the angle at
Let the internal bisector of the angle at  meet the side
meet the side  at
at  and the line
and the line  at
at  and let the internal bisector of the angle at
and let the internal bisector of the angle at  meet the side
meet the side  at
at  and the line
and the line  at
at  If
If 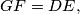 prove that
prove that 
 Školjka
Školjka 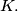 The midpoint of
The midpoint of  and that of
and that of  The lines
The lines 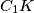 and
and 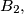 the lines
the lines  and
and 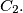 If the areas of the triangles
If the areas of the triangles 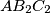 and
and 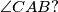
 ,
,  be the centroid, the incenter and the orthocenter of triangle
be the centroid, the incenter and the orthocenter of triangle 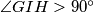 .
. 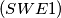 Six points
Six points 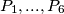 are given in
are given in 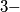 dimensional space such that no four of them lie in the same plane. Each of the line segments
dimensional space such that no four of them lie in the same plane. Each of the line segments 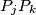 is colored black or white. Prove that there exists one triangle
is colored black or white. Prove that there exists one triangle 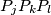 whose edges are of the same color.
whose edges are of the same color.  and
and  are in the sides
are in the sides 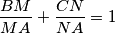 , then prove that the centroid of
, then prove that the centroid of  .
. 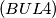 Let
Let 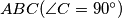 such that
such that 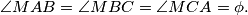 Let
Let 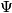 be the acute angle between the medians of
be the acute angle between the medians of 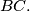 Prove that
Prove that 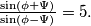
 Let
Let 
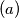 Prove that all conics passing through the points
Prove that all conics passing through the points 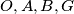 are hyperbolas.
are hyperbolas.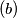 Find the locus of the centers of these hyperbolas.
Find the locus of the centers of these hyperbolas.