Slični zadaci
Determine the smallest positive real number  with the following property. Let
with the following property. Let  be a convex quadrilateral, and let points
be a convex quadrilateral, and let points  ,
,  ,
,  , and
, and  lie on sides
lie on sides  ,
,  ,
,  , and
, and  , respectively. Consider the areas of triangles
, respectively. Consider the areas of triangles 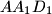 ,
,  ,
, 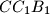 and
and 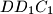 ; let
; let  be the sum of the two smallest ones, and let
be the sum of the two smallest ones, and let  be the area of quadrilateral
be the area of quadrilateral 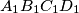 . Then we always have
. Then we always have 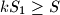 .
.
Author: unknown author, USA
 with the following property. Let
with the following property. Let  be a convex quadrilateral, and let points
be a convex quadrilateral, and let points  ,
,  ,
,  , and
, and  lie on sides
lie on sides  ,
,  ,
,  , and
, and  , respectively. Consider the areas of triangles
, respectively. Consider the areas of triangles 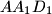 ,
,  ,
, 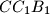 and
and 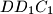 ; let
; let  be the sum of the two smallest ones, and let
be the sum of the two smallest ones, and let  be the area of quadrilateral
be the area of quadrilateral 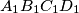 . Then we always have
. Then we always have 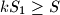 .
. Author: unknown author, USA
Let the sides  and
and  of the quadrilateral
of the quadrilateral  (such that
(such that  is not parallel to
is not parallel to  ) intersect at point
) intersect at point  . Points
. Points 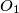 and
and 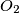 are circumcenters and points
are circumcenters and points 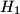 and
and 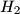 are orthocenters of triangles
are orthocenters of triangles 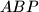 and
and 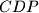 , respectively. Denote the midpoints of segments
, respectively. Denote the midpoints of segments  and
and 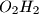 by
by 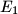 and
and 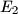 , respectively. Prove that the perpendicular from
, respectively. Prove that the perpendicular from 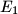 on
on  , the perpendicular from
, the perpendicular from 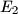 on
on  and the lines
and the lines 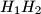 are concurrent.
are concurrent.
Proposed by Ukraine
 and
and  of the quadrilateral
of the quadrilateral  (such that
(such that  is not parallel to
is not parallel to  ) intersect at point
) intersect at point  . Points
. Points 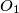 and
and 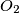 are circumcenters and points
are circumcenters and points 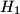 and
and 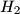 are orthocenters of triangles
are orthocenters of triangles 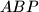 and
and 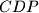 , respectively. Denote the midpoints of segments
, respectively. Denote the midpoints of segments  and
and 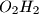 by
by 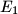 and
and 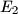 , respectively. Prove that the perpendicular from
, respectively. Prove that the perpendicular from 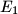 on
on  , the perpendicular from
, the perpendicular from 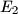 on
on  and the lines
and the lines 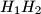 are concurrent.
are concurrent.Proposed by Ukraine
 Školjka
Školjka  in the plane, we define
in the plane, we define 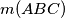 to be the smallest length of the three heights of the triangle
to be the smallest length of the three heights of the triangle  , where in the case
, where in the case  ,
,  ,
,  are collinear, we set
are collinear, we set 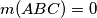 . Let
. Let  in the plane,
in the plane, 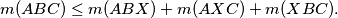
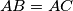 .
.  is the midpoint of
is the midpoint of  is the point on the line
is the point on the line  such that
such that 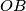 is perpendicular to
is perpendicular to  is an arbitrary point on
is an arbitrary point on  lies on the line
lies on the line  lies on the line
lies on the line  such that
such that  are distinct and collinear. Prove that
are distinct and collinear. Prove that  is perpendicular to
is perpendicular to  if and only if
if and only if  .
.  ,
,  ,
,  meet the sides
meet the sides  ,
,  ,
, 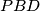 ,
, 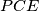 ,
, 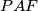 are all equal. Prove that each of these areas is equal to the area of triangle
are all equal. Prove that each of these areas is equal to the area of triangle 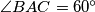 . Let
. Let  and let
and let 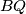 bisect
bisect 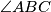 , with
, with 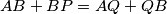 , what are the angles of the triangle?
, what are the angles of the triangle? 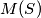 and
and 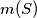 denote the greatest and smallest areas, respectively, of triangles determined by three points from
denote the greatest and smallest areas, respectively, of triangles determined by three points from  ?
?