Slični zadaci
A circle  with center
with center 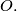 and a line
and a line  which does not touch circle
which does not touch circle 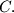
 is perpendicular to
is perpendicular to 
 is on
is on 
 is on
is on  draw two tangents
draw two tangents 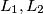 to circle
to circle 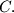
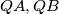 are perpendicular to
are perpendicular to 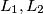 respectively. (
respectively. ( on
on 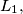
 on
on 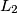 ). Prove that, line
). Prove that, line  intersect
intersect  at a fixed point.
at a fixed point.
Original formulation:
A line does not meet a circle
does not meet a circle  with center
with center 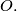
 is the point on
is the point on  such that
such that 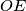 is perpendicular to
is perpendicular to 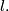
 is any point on
is any point on  other than
other than 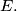 The tangents from
The tangents from  to
to  touch it at
touch it at  and
and 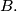
 is the point on
is the point on 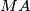 such that
such that 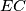 is perpendicular to
is perpendicular to 
 is the point on
is the point on 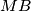 such that
such that  is perpendicular to
is perpendicular to 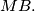 The line
The line  cuts
cuts 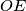 at
at 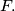 Prove that the location of
Prove that the location of  is independent of that of
is independent of that of 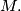
 with center
with center 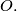 and a line
and a line  which does not touch circle
which does not touch circle 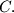
 is perpendicular to
is perpendicular to 
 is on
is on 
 is on
is on  draw two tangents
draw two tangents 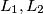 to circle
to circle 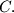
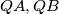 are perpendicular to
are perpendicular to 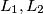 respectively. (
respectively. ( on
on 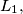
 on
on 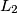 ). Prove that, line
). Prove that, line  intersect
intersect  at a fixed point.
at a fixed point. Original formulation:
A line
 does not meet a circle
does not meet a circle  with center
with center 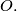
 is the point on
is the point on  such that
such that 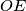 is perpendicular to
is perpendicular to 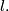
 is any point on
is any point on  other than
other than 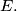 The tangents from
The tangents from  to
to  touch it at
touch it at  and
and 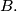
 is the point on
is the point on 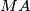 such that
such that 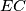 is perpendicular to
is perpendicular to 
 is the point on
is the point on 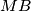 such that
such that  is perpendicular to
is perpendicular to 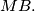 The line
The line  cuts
cuts 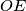 at
at 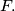 Prove that the location of
Prove that the location of  is independent of that of
is independent of that of 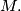
Let  be a fixed convex quadrilateral with
be a fixed convex quadrilateral with 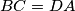 and
and  not parallel with
not parallel with  . Let two variable points
. Let two variable points  and
and  lie of the sides
lie of the sides  and
and  , respectively and satisfy
, respectively and satisfy 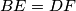 . The lines
. The lines  and
and  meet at
meet at  , the lines
, the lines  and
and  meet at
meet at  , the lines
, the lines  and
and  meet at
meet at  .
.
Prove that the circumcircles of the triangles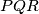 , as
, as  and
and  vary, have a common point other than
vary, have a common point other than  .
.
 be a fixed convex quadrilateral with
be a fixed convex quadrilateral with 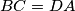 and
and  not parallel with
not parallel with  . Let two variable points
. Let two variable points  and
and  lie of the sides
lie of the sides  and
and  , respectively and satisfy
, respectively and satisfy 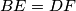 . The lines
. The lines  and
and  meet at
meet at  , the lines
, the lines  and
and  meet at
meet at  , the lines
, the lines  and
and  meet at
meet at  .
. Prove that the circumcircles of the triangles
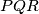 , as
, as  and
and  vary, have a common point other than
vary, have a common point other than  .
.  Školjka
Školjka  and
and  touch internally the circle
touch internally the circle  in M and N and the center of
in M and N and the center of  be the incenter of triangle
be the incenter of triangle  . Let
. Let 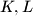 and
and 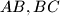 and
and  , respectively. The line
, respectively. The line  passes through
passes through 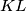 . The lines
. The lines 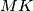 and
and 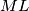 intersect
intersect  . Prove that
. Prove that 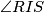 is acute.
is acute. 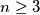 be a positive integer. Let
be a positive integer. Let 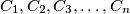 be unit circles in the plane, with centres
be unit circles in the plane, with centres 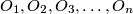 respectively. If no line meets more than two of the circles, prove that
respectively. If no line meets more than two of the circles, prove that 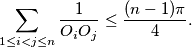
 and
and 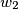 with centres
with centres 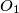 and
and  are externally tangent at point
are externally tangent at point  at points
at points  are on the same side of
are on the same side of 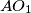 ,
, 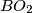 ,
, 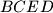 is a cyclic quadrilateral. Let
is a cyclic quadrilateral. Let 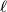 be a line passing through
be a line passing through 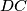 at
at  . Suppose also that
. Suppose also that 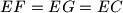 . Prove that
. Prove that  .
.