Slični zadaci
Show that for any finite set  of distinct positive integers, we can find a set
of distinct positive integers, we can find a set  ⊇
⊇  such that every member of
such that every member of  divides the sum of all the members of
divides the sum of all the members of  .
.
Original Statement:
A finite set of (distinct) positive integers is called a DS-set if each of the integers divides the sum of them all. Prove that every finite set of positive integers is a subset of some DS-set.
 of distinct positive integers, we can find a set
of distinct positive integers, we can find a set  ⊇
⊇  such that every member of
such that every member of  divides the sum of all the members of
divides the sum of all the members of  .
. Original Statement:
A finite set of (distinct) positive integers is called a DS-set if each of the integers divides the sum of them all. Prove that every finite set of positive integers is a subset of some DS-set.
Ten points are marked in the plane so that no three of them lie on a line. Each pair of points is connected with a segment. Each of these segments is painted with one of  colors, in such a way that for any
colors, in such a way that for any  of the ten points, there are
of the ten points, there are  segments each joining two of them and no two being painted with the same color. Determine all integers
segments each joining two of them and no two being painted with the same color. Determine all integers  ,
,  , for which this is possible.
, for which this is possible.
 colors, in such a way that for any
colors, in such a way that for any  of the ten points, there are
of the ten points, there are  segments each joining two of them and no two being painted with the same color. Determine all integers
segments each joining two of them and no two being painted with the same color. Determine all integers  ,
,  , for which this is possible.
, for which this is possible. Let 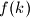 be the number of all non-negative integers
be the number of all non-negative integers  satisfying the following conditions:
satisfying the following conditions:
(1) The integer has exactly
has exactly  digits in the decimal representation (where the first digit is not necessarily non-zero!), i. e. we have
digits in the decimal representation (where the first digit is not necessarily non-zero!), i. e. we have  .
.
(2) These digits of n can be permuted in such a way that the resulting number is divisible by 11.
digits of n can be permuted in such a way that the resulting number is divisible by 11.
Show that for any positive integer number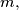 we have
we have  .
.
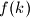 be the number of all non-negative integers
be the number of all non-negative integers  satisfying the following conditions:
satisfying the following conditions: (1) The integer
 has exactly
has exactly  digits in the decimal representation (where the first digit is not necessarily non-zero!), i. e. we have
digits in the decimal representation (where the first digit is not necessarily non-zero!), i. e. we have  .
. (2) These
 digits of n can be permuted in such a way that the resulting number is divisible by 11.
digits of n can be permuted in such a way that the resulting number is divisible by 11. Show that for any positive integer number
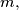 we have
we have  .
.  Školjka
Školjka  and
and  be relatively prime positive integers. A subset
be relatively prime positive integers. A subset 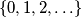 is called ideal if
is called ideal if 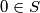 and for each element
and for each element 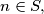 the integers
the integers 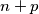 and
and 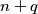 belong to
belong to 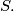 Determine the number of ideal subsets of
Determine the number of ideal subsets of 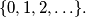
 and
and  be odd integers so that
be odd integers so that  . Show that there are two integers of the same colour whose difference has one of the following values:
. Show that there are two integers of the same colour whose difference has one of the following values: 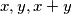 or
or  .
.  Find the least number
Find the least number  for which it is possible to place
for which it is possible to place  chessboard so that no column or row contains a block of
chessboard so that no column or row contains a block of 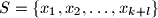 be a
be a 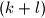 -element set of real numbers contained in the interval
-element set of real numbers contained in the interval ![[0, 1]](/media/m/7/f/0/7f04c41d91b0555ac792351e836cbd4e.png) ;
;  are positive integers. A
are positive integers. A 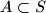 is called nice if
is called nice if 
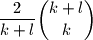 .
.