1. Let

be an acute-angled triangle with
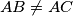
. The circle with diameter

intersects the sides

and

at

and

respectively. Denote by

the midpoint of the side

. The bisectors of the angles

and
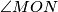
intersect at

. Prove that the circumcircles of the triangles
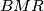
and
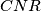
have a common point lying on the side

.
%V0
1. Let $ABC$ be an acute-angled triangle with $AB\neq AC$. The circle with diameter $BC$ intersects the sides $AB$ and $AC$ at $M$ and $N$ respectively. Denote by $O$ the midpoint of the side $BC$. The bisectors of the angles $\angle BAC$ and $\angle MON$ intersect at $R$. Prove that the circumcircles of the triangles $BMR$ and $CNR$ have a common point lying on the side $BC$.