Slični zadaci
A positive integer  is called balanced, if
is called balanced, if 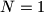 or if
or if  can be written as a product of an even number of not necessarily distinct primes. Given positive integers
can be written as a product of an even number of not necessarily distinct primes. Given positive integers  and
and  , consider the polynomial
, consider the polynomial  defined by
defined by 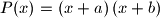 .
.
a) Prove that there exist distinct positive integers and
and  such that all the number
such that all the number 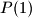 ,
, 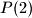 , ...,
, ..., 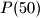 are balanced.
are balanced.
b) Prove that if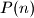 is balanced for all positive integers
is balanced for all positive integers  , then
, then 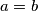 .
.
Proposed by Jorge Tipe, Peru
 is called balanced, if
is called balanced, if 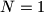 or if
or if  can be written as a product of an even number of not necessarily distinct primes. Given positive integers
can be written as a product of an even number of not necessarily distinct primes. Given positive integers  and
and  , consider the polynomial
, consider the polynomial  defined by
defined by 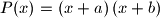 .
.a) Prove that there exist distinct positive integers
 and
and  such that all the number
such that all the number 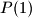 ,
, 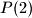 , ...,
, ..., 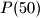 are balanced.
are balanced.b) Prove that if
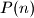 is balanced for all positive integers
is balanced for all positive integers  , then
, then 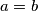 .
.Proposed by Jorge Tipe, Peru
 Školjka
Školjka  Odredi sve proste brojeve
Odredi sve proste brojeve 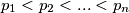 takve da je
takve da je 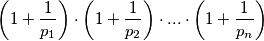 prirodan broj.
prirodan broj.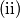 Postoje li prirodni brojevi
Postoje li prirodni brojevi 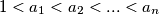 takvi da je
takvi da je 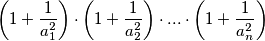 prirodan broj.
prirodan broj. 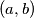 such that
such that  divides
divides 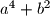 .
.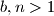 be integers. Suppose that for each
be integers. Suppose that for each  there exists an integer
there exists an integer 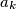 such that
such that 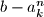 is divisible by
is divisible by 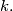 Prove that
Prove that 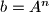 for some integer
for some integer 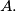
 be a prime number. Prove that if
be a prime number. Prove that if  are integers (not necessarily positive) satisfying the equations
are integers (not necessarily positive) satisfying the equations 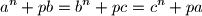
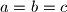 .
.  ,
, 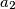 ,
,  ,
,  be distinct positive integers,
be distinct positive integers,  . Prove that there exist distinct indices
. Prove that there exist distinct indices  and
and  such that
such that 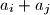 does not divide any of the numbers
does not divide any of the numbers 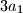 ,
,  ,
, 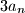 .
.