On a blackboard there are
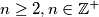
numbers. In each step we select two numbers from the blackboard and replace both of them by their sum. Determine all numbers

for which it is possible to yield

identical number after a finite number of steps.
%V0
On a blackboard there are $n \geq 2, n \in \mathbb{Z}^{+}$ numbers. In each step we select two numbers from the blackboard and replace both of them by their sum. Determine all numbers $n$ for which it is possible to yield $n$ identical number after a finite number of steps.