Let

,

,

be real numbers satisfying
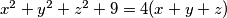
. Prove that
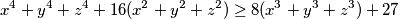
and determine when equality holds.
%V0
Let $x$, $y$, $z$ be real numbers satisfying $x^2+y^2+z^2+9=4(x+y+z)$. Prove that $$x^4+y^4+z^4+16(x^2+y^2+z^2) \ge 8(x^3+y^3+z^3)+27$$ and determine when equality holds.