Let

be positive real numbers such that
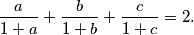
Prove that
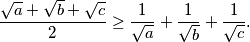
%V0
Let $a, b, c$ be positive real numbers such that $$\frac{a}{1+a}+\frac{b}{1+b}+\frac{c}{1+c}=2\text{.}$$
Prove that $$\frac{\sqrt a + \sqrt b+\sqrt c}{2} \geq \frac{1}{\sqrt a}+\frac{1}{\sqrt b}+\frac{1}{\sqrt c}\text{.}$$